Which Describes the Combined Variation in the Formula

Set up 2 variation equations the first using k_ 1 and the second k_ 2 as constants. Here y varies jointly as x and z.

Which Describes The Combined Variation In The Formula H V Pie R2 Brainly Com

V varies inversely with a h and r2.

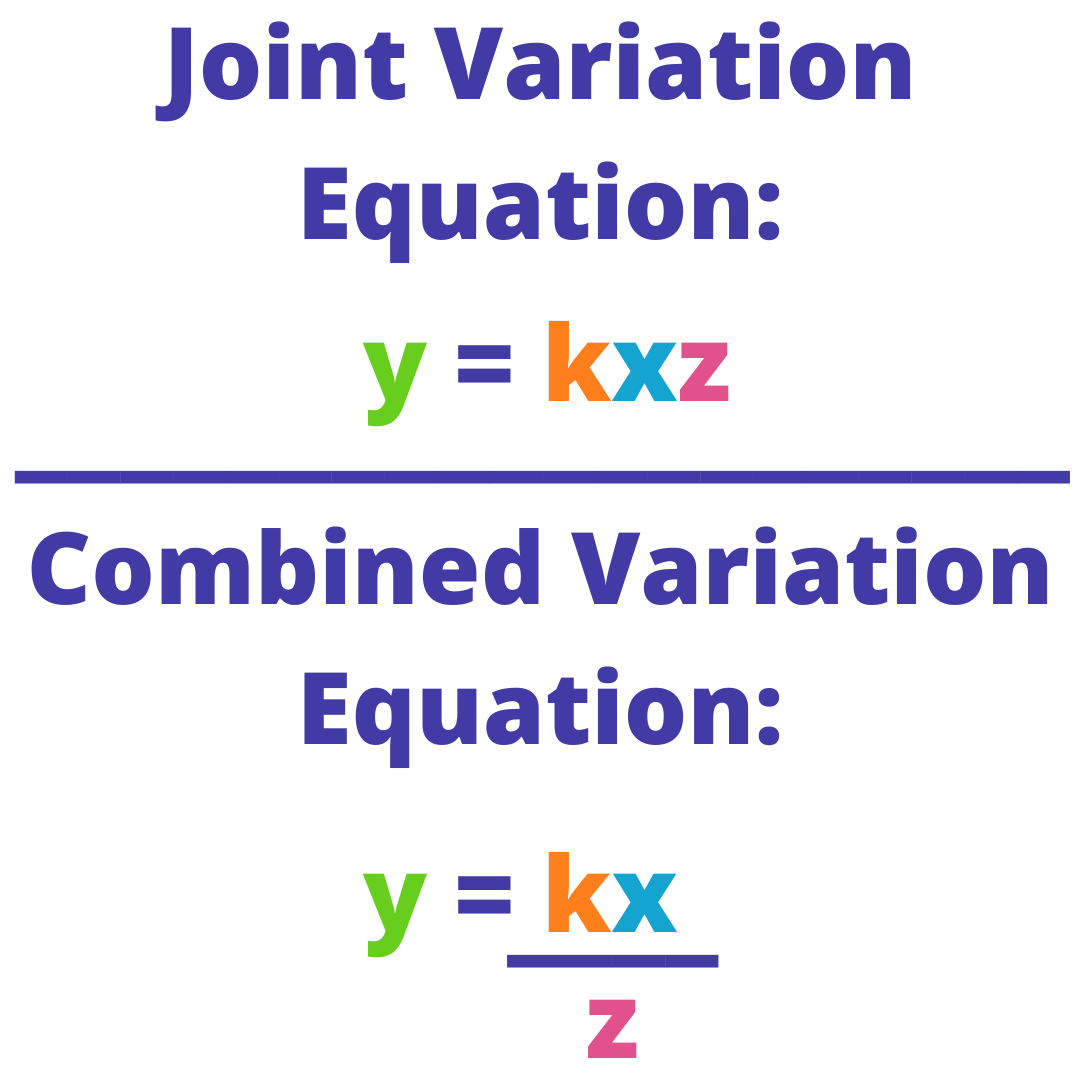
. Up to 10 cash back Combined variation describes a situation where a variable depends on two or more other variables and varies directly with some of them and varies inversely with others when the rest of the variables are held constant. K y x 3 25 2 3 25 8 k y x 3 25 2 3 25 8. V Bh 3.

Z k x y. V and l or V and w or V and h are all directly related. Membership Math Anxiety Sudoku Biographies of Mathematicians CPC Podcast.

Which describes the combined variation in the formula v ℓwh. In Algebra sometimes we have functions that vary in more than one element. Z k x y.

Y 25 8 x 3 y 25 8 x 3. This means when the price goes up instead of demand decreasing demand actually. HfracVpi r2.

Pick a well-known formula and describe the type of variation as well as the constant of variation k. In other words F is related to m. The general formula for direct variation with a cube is y k x 3 y k x 3.

V varies inversely with ℓ w and h. More Examples on Joint Variation. Up to 10 cash back Joint Variation.

V varies directly with h. The equations expressing combined variation take the form x kyz. This is the concept of proportionality.

B and h vary inversely. With prestige pricing elasticity in demand can be a positive number. We can use the example of John Ray.

Now use the constant to write an equation that represents this relationship. The force of attraction F of a body varies directly as its mass m times a constant k and inversely as the square of the distance d between the bodies. Vlwh-----Ive never hear of combined variation but here goes.

Joint variation describes a situation where one variable depends on two or more other variables and varies directly as each of them when the others are held constant. With combined variation we have both direct variation and indirect. Y kx where k is the constant of variation and k 0 y kxz represents joint variation.

Describe the combined variation that is modeled by each formula. Correct answer to the question Which describes the combined variation in the formula V xr2h. V varies directly with B.

V varies directly with a h and r2. You can put this solution on YOUR website. A company uses the _____ pricing strategy when it sells a popular item at an artificially low price.

Combined variation is a variation where a quantity depends on two or more other quantities and varies directly with some of them and varies inversely with others. R2 and h vary inversely. When this happens we say that the functions have joint variation or combined variation.

Perhaps the most important historical discovery of this type of combined variation is Newtons law of universal gravitation which states that the gravitation force of attraction F between two particles is jointly proportional to their masses m and M and inversely proportional to the square of the distance d between them. And when -- What is given. The area A of a triangle with base b and height h is given by the formula A½bh.

The constant can be found by dividing y y by the cube of x x. V varies jointly with ℓ w and h. V varies directly with r2.

D f rt rt where d. Correct answer to the question. A V varies jointly as lw and h.

Therefore the correct answer is. It is a variation in which the quotient of the product of two variables and a variable is a constant. Describe the combined variation that is modeled by each formula.

Enter Joint Variation Parameters. Correct answer - Which describes the combined variation in the formula V 314r2n. Varies jointly with.

V varies jointly with h and 2. For some constant k. Describe the combined variation that is modeled by each formula.

P f lw 2l 2w where P is the perimeter and is a function of width and length. So the area of a triangle varies jointly as the base and height both direct with the constant of variation ½. The function notation of the formulas can be written as.

Joint Variation Equations Video. When one goes up in value the other goes down. Joint variation is a variation where a quantity varies directly as the product of two or more other quantities.

When one goes up the other goes up-----l and w or l and h or w and h are all indirectly related. Joint Variation Equations Calculator. We are required to find the combined variation for the volume formula given by.

Answer by stanbon 75887 Show Source. The first equation is inverse variation and the second equation is direct variation. Example 1 If y varies.

In respect to this how do you solve combined variations step by step. Vlengthwidthheight Vlwh here we see that volume is directly proportional to width length and height. Joint variation is direct variation to more than one variable for example d r t.

We say z varies jointly as x and y if. Direct variation between variables x and y can be expressed as. V varies directly with h.

Combined variation is a kind of variation which involves both direct and inverse variations. V f rh Πr²h where V is the volume and is a function of radius and height. For example if z varies directly as x and inversely as y we have the following combined variation equation.

V varies inversely with h and 12. Y 7xz here y varies jointly as x and z y 7x 2 z 3 here y varies jointly as x 2 and z 3 Area of a triangle is an example of joint variation. Solve for k_ 1 and k_ 2 separately using the fact that y and z are both 12 when x3.

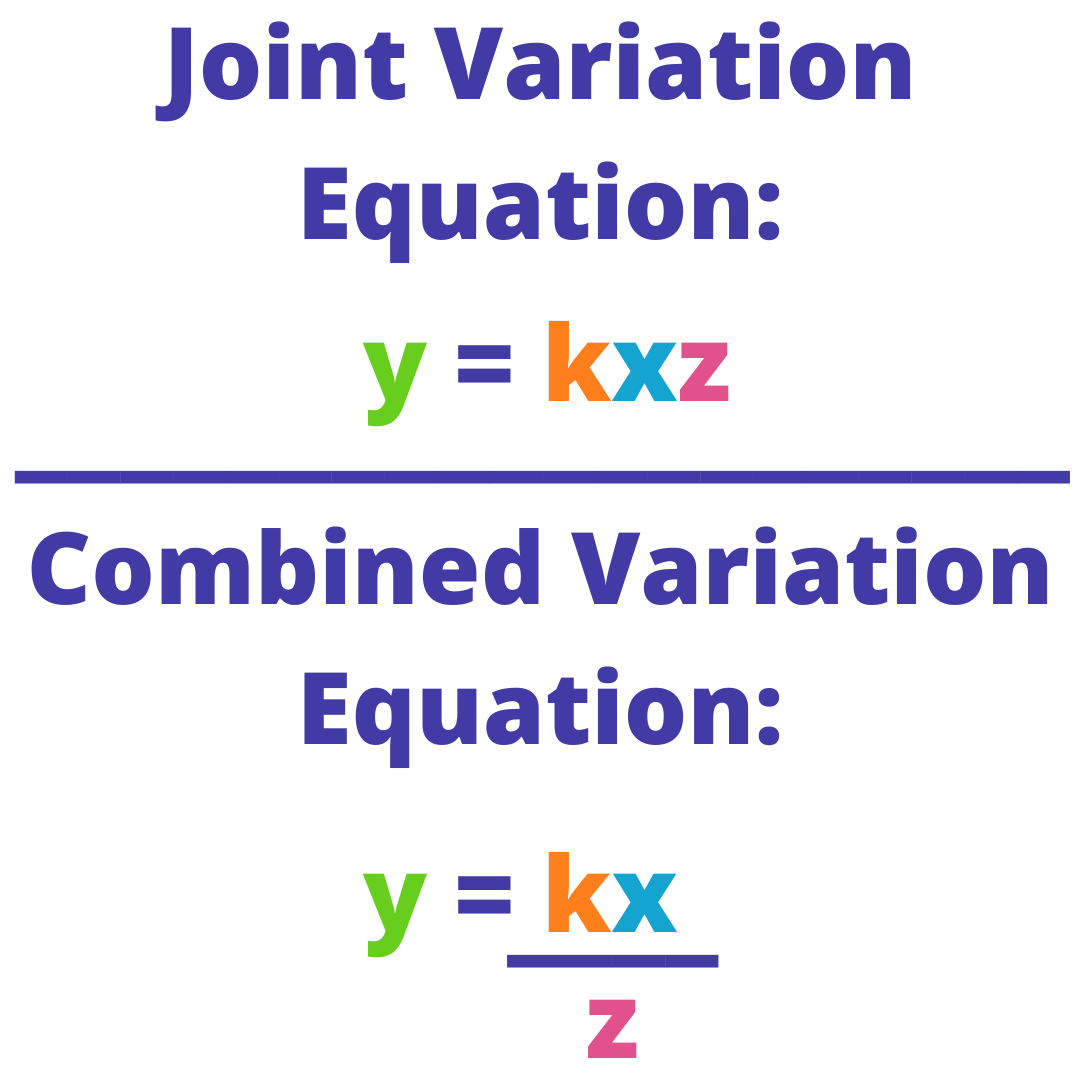
Joint Variation And Combined Variation Definitions Expii

Joint Or Combined Variation Video Lessons Examples And Solutions

Solved 6 V Which Describes The Combined Variation In The Chegg Com